The Possibility that All Life is a Single 5^th-Dimensional Entity

Mike Blaber

11/9/06

The relationship between time and the perception higher-order dimensions

Example 1:  A one dimensional creature and its ability to perceive a two-dimensional object

Imagine that there is a one-dimensional creature (Walter).  He lives in a one-dimensional world (a line), and can conceive only of one-dimensional objects (i.e. something that has variable length, but no width or height).  The concepts of width and height have no meaning to Walter.

.

How might Walter perceive a two-dimensional object, such as a circle?  It is not fair to ask him to simply imagine a circle; being a one-dimensional creature, the idea of perceiving in two-dimensions would be an utterly foreign concept, and his one-dimensional brain may not even be capable of constructing such a reality.

However, if we passed a circle through his one dimensional world, here is how it might look to us:

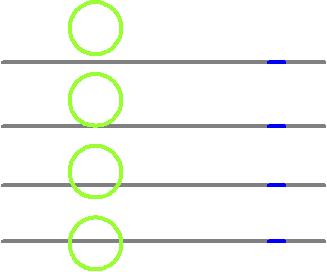
Since Walter cannot see beyond his one-dimensional world, here is how the circle (a two-dimensional object) would appear as it passed through his world:

Thus, to Walter a “circle” is comprehended as something that suddenly appears as a dot, then splits into two dots that move in opposite directions equidistant to the starting point, and then move back, converging once again to a dot, and then it disappears completely. 

There are various ways to pervert the little guy’s definition of a circle – we could start to insert the circle into his world and then withdraw it, we could start to insert it with an initial velocity, and then change the velocity, we could insert it at an angle, etc.  We could even be so perverse as to consistently do these different things to different one-dimensional creatures, so that each one had a different definition of what a circle was (sort of like the three blind men examining different parts of an elephant an arriving at different conclusions as to what an elephant was). 

In any case, let’s not be mean, and instead, always pass the circle through at a constant velocity and normal to Walter’s world.  In this case, he (or one of his more clever friends) could develop a mathematical expression for the circle that would accurately describe the circles behavior over time, and in this way, Walter and his friends could “comprehend” what this two-dimensional object.  Thus, as far as Walter and his friends are concerned, a circle is a one-dimension object that changes over time (in this case, the property of appearing, splitting into two, moving apart at a predictable speed, stopping, moving back together at a predictable speed, and then coalescing into a single point, and the disappearing from their world entirely.

Example 2: A two-dimensional creature and its ability to perceive a three-dimensional object

Assume that Walter is a two-dimensional creature: he has length and width but no height.  Again, he has no comprehension of “height”, and it is possible that his two-dimensional brain is simply incapable of conceiving of this additional dimension.

Walter is now capable of perceiving and comprehending a circle. It can exist entirely within his world at any given instant:

How would two-dimensional Walter perceive a three-dimensional object like a hollow sphere?

Once again, we could pass it completely through his world so that he can perceive it in it’s entirety:

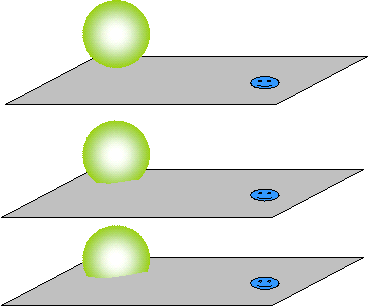
And to Walter it would look like this:

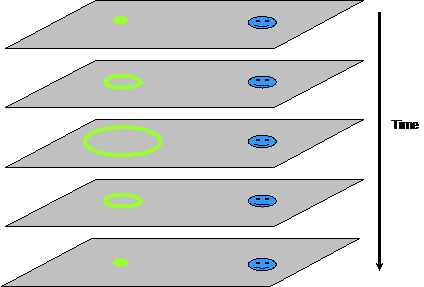
Thus, a three-dimensional object (sphere) can be comprehended by two-dimensional Walter as a two-dimensional object that changes over time:  to Walter, a “sphere” suddenly makes its appearance in his world as a dot which over time becomes an ever-expanding circle; this expansion slows down, stops and reverses, becomes a dot, then disappears from his world.

Example 3: Now we come to three-dimensional Walter, something we are familiar with.  He lives in a three-dimensional world and he has length, width and height:

Walter can comprehend a three-dimensional object like a sphere, it can exist entirely within his world:

How would a fourth-dimensional object appear to Walter?  We cannot draw a fourth-dimensional object; however, we can predict how such an object might look to Walter:

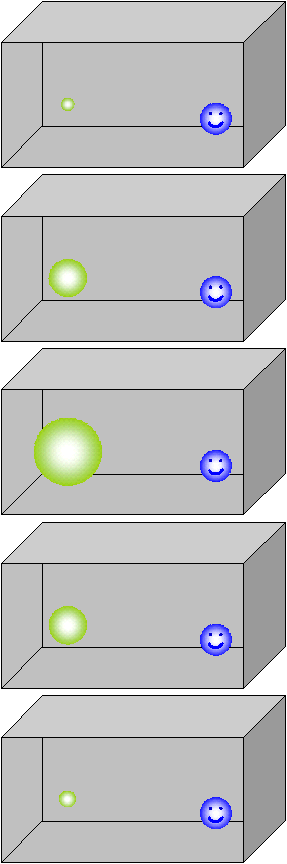
In this case, some fourth-dimensional object that appears to be related in some way to what we know as a sphere, suddenly appears as a dot in our world, then expands, slows down, stops, and then contracts to a dot, and then suddenly disappears.  Although it appears to change over time, it is actually a single entity (fourth-dimensional) and so the change over time in three-dimensions is the only way that we (being three-dimensional beings) can comprehend it.

Therefore, it is entirely possible that is an object in our three-dimensional world is observed to change over time (particularly if the change is observed to be a predictable change), that it is actually a single fourth-dimensional object (and understandable to us only by observing over time).  Here is one possible example of such a fourth-dimensional object:

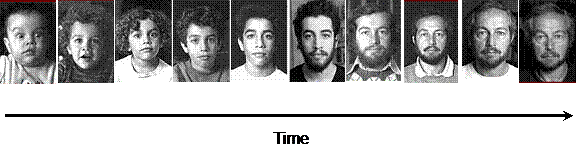
It is possible that this person is simultaneously all these stages of life, but can only be understood by us (and him; in our three dimensional world) through the passage of time.  His appearance and disappearance in “life” marking the entrance and exit of this fourth dimensional entity through our three-dimensional world.

Example 4: A fifth dimension…

How would a fifth dimensional object appear in a fourth dimensional world?  This is too weird.  But, we could ask how a fifth dimensional object appears in a three dimensional world.  It is analogous to how a three dimensional object appears in a one dimensional world.  How would a hollow sphere (three-dimensions) appear to one-dimensional Walter?  The answer is, it depends on how the sphere is positioned as it goes through Walter’s one-dimensional world.  If just the edge or cusp of the sphere clips Walter’s world, then the sphere appears as a dot, barely separates into two points, immediately coalesces back to a dot, and disappears.  If the sphere is moved over slightly, and then passed through Walter’s one-dimensional world again, the dots appear to separate further before reversing direction, coalescing and disappearing.  So, it seems that the sphere (three-dimensions) is perceived in a one-dimensional world as change-with-time (the circle) that itself changes with time (i.e. each passage yields different behavior of the circle).  Thus, we conclude that a fifth dimensional object passing through a three-dimensional world would manifest itself as a three-dimensional object whose change with time itself changes with time.  Is there an example of this seemingly complex behavior of an object in three-dimensions?  In the case of the three-dimensional object above (i.e. a person, that clearly changes with time, and is therefore potentially a fourth dimensional object), there is:

The entity above (homo sapiens) did not exist 1 million years ago.  If humans were immutable (as a species) but changed with time (“aged”) they could conceivably have no higher complexity than four dimensions; however, in addition to individuals aging with time, the species has also changed with time.  Thus, this change with change in time is a characteristic of a fifth-dimensional object passing through (or being perceived within) a three-dimensional world.  In this case, what is the fifth-dimensional object?  It appears to be an entity that simultaneously includes the species above.  But the past extends beyond the 5 million years ago shown in the picture, thus, other precursor species are potentially included in the single fifth-dimensional object.  This would appear to include, therefore, all life.  Thus, it is possible that all life, past, present and future, is a single fifth-dimensional object.